This chapter covers the calculation of the surface area and volume of selected solid figures.

EO 1.5

Given the formula, CALCULATE the volume and surface areas of the following solid figures:

a. Rectangular solid

b. Cube

c. Sphere

d. Right circular cone

e. Right circular cylinder

The three flat shapes of the triangle, rectangle, and circle may become solids by adding the third dimension of depth. The triangle becomes a cone;

circle, a cylinder.

the rectangle, a rectangular solid; and the

Rectangular Solids

A rectangular solid is a six-sided solid figure with faces that are rectangles, as shown in Figure 14.

The volume of a rectangular solid is calculated using the following formula:

V = abc (3-11)

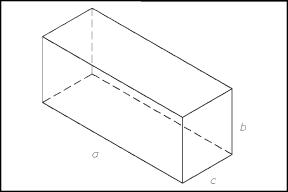
Figure 14 Rectangular Solid

The surface area of a rectangular solid is calculated using the following formula:

SA = 2(ab + ac + bc) (3-12)

The surface area of a rectangular solid is expressed in square units, and the volume of a rectangular solid is expressed in cubic units.

Example:

Calculate the volume and surface area of a rectangular solid with a = 3", b = 4", and c = 5". Be sure to include units in your answer.

Solution:

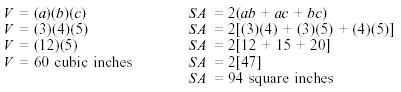
Cube

A cube is a six-sided solid figure whose faces are congruent squares, as shown in Figure 15.

The volume of a cube is calculated using the following formula:

The surface area of a cube is calculated using the following formula:

SA = 6a² (3-14)

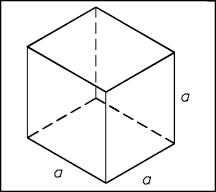
Figure 15 Cube

The surface area of a cube is expressed in square units, and the volume of a cube is expressed in cubic units.

Example:

Calculate the volume and surface area of a cube with a = 3". Be sure to include units in your answer.

Solution: