Trigonometric Functions

When the value of a trigonometric function of an angle is known, the size of the angle can be found. The inverse trigonometric function, also known as the arc function, defines the angle based on the value of the trigonometric function. For example, the sine of 21 equals 0.35837; thus, the arc sine of 0.35837 is 21.

There are two notations commonly used to indicate an inverse trigonometric function.

The notation arcsin means the angle whose sine is. The notation arc can be used as a prefix to any of the trigonometric functions. Similarly, the notation sin^-' means the angle whose sine is. It is important to remember that the -1 in this notation is not a negative exponent but merely an indication of the inverse trigonometric function.

To perform this function on a calculator, enter the numerical value, press the INV key, then the SIN, COS, or TAN key. To calculate the inverse function of cot, csc, and sec, the reciprocal key must be pressed first then the SIN, COS, or TAN key.

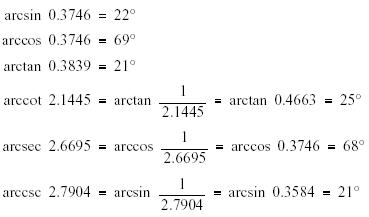
Examples:

Evaluate the following inverse trigonometric functions.

Summary

The important information in this chapter is summarized below.

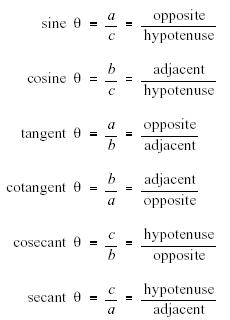
Trigonometric Functions Summary

The six trigonometric functions are: