|
LINE TANGENT TO A CIRCLE
AT
A GIVEN POINT A line that is tangent to a circle at a given point
is
perpendicular to the radius that intersects the point.
It follows that one method of drawing a line
tangent to a circle at a given point is to draw the
radius that intersects the point, and then draw the
line tangent at the point of intersection and perpendicular
to the radius.
Another method is shown in figure 4-32. To draw
a line tangent to the circle at P, set a compass
to the radius of the circle, and, with P as
a center, strike an arc that intersects the circle at
A. With the compass still set to the radius of the
circle, use A as a center and strike an arc that intersects
the first arc at B. With B as a center and
the compass still set to the radius of the circle,
strike another arc. A line through the point of
intersection (0) of the last drawn arc and through
P is tangent to the circle at P.
CIRCULAR ARC OF A GIVEN
RADIUS TANGENT TO TWO
STRAIGHT LINES
Drawing a fillet or round comprises the problem
of drawing a circular arc of a given radius tangent
to two nonparallel lines.
Figure 4-33 shows a method that can be used when
the two nonparallel lines form a right angle. AB
is the given radius of the arc. Set a compass to
this radius, and, with the point of intersection of
the lines as a center, strike an arc intersecting the
lines at C and D. With C and D as centers and
the same radius, strike intersecting arcs as
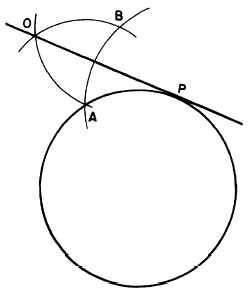
Figure 4-32.-Line tangent to a given point on a circle.
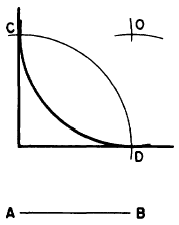
Figure 4-33.-Circular arc tangent to two lines that form a
right angle.
shown. The point of intersection of these arcs (0) is
the center of the circle of which an arc of the given
radius is tangent to the lines.
Figure 4-34 shows a method that can be used regardless
of the size of the angle formed by the lines.
Again AB equals the given radius of the arc, and
the problem is to draw an arc with radius equal
to AB, tangent to CD and EF. Draw GH parallel
to CD and at a distance from CD equal to
the given radius of the arc. Draw IJ parallel to
EF and also at a distance equal to the given radius
of the arc. The point of intersection between
GH and IJ (P) is the center of the circle of
which an arc of the given radius is tangent to CD
and EF.
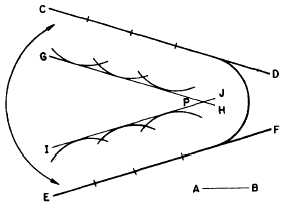
Figure 4-34.-Circular arc tangent to two lines that form
any
angle.
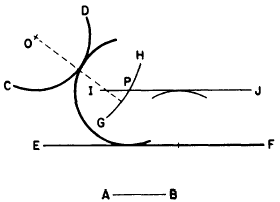
Figure 4-35.-Circular arc tangent to a straight line and another
circular arc.
|


|

