Under "Tape Corr." in the fifth column, the standard error for each interval is entered. Again, the tape had a standard error of 0.013 ft per 100 ft; therefore, the standard error for each interval except the last is 0.013 ft. For the last interval of 73.18 ft, the error works out as 0.009 ft.

If you will look to the right of the "Tape Corr." column, you will see the "Temp. Corr." column. For the first two intervals measured, the temperature of the tape was 78F, or 10F above standard. The correction amounts to 0.01 ft for each 15F above standard; therefore, the total temperature correction for each of these intervals equals the value of x in the equation

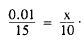
The total temperature correction is 0.007 ft. Because the temperature was above standard, the tape lengthened and was reading short. So the

corrections should be added as indicated by the plus signs.

To the right of the "Temp. Corr." column is the "Slope Corr." column. Its entries are to be subtracted as indicated. Use the following equation to compute the slope correction. 

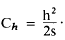
For the first taped interval, we have an h of 6.0 ft and an s of 100 ft.

Therefore

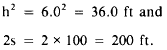
The slope correction is computed as follows:

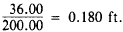
Next to the column for slope correction comes the "Total Corr." column, containing the algebraic sum of the three corrections for each taped interval. Finally, in the "Horiz. Dist." column, each value is determined by subtracting the total correction for each interval from the measured slope distance for that interval. (This example used in figure 12-17 happens to be all negative.)

At the bottom of this column, the sum of the horizontal distances appears. This is the horizontal distance from station K to station L.