Before the modern instruments used to measure angles directly in the field were devised, the tape (or rather, its equivalent, the Gunters chain) was often used. This tape was used not only for measuring linear distances but also for measuring angles more accurately than was possible with a compass.

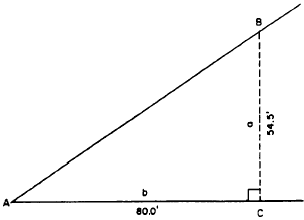
Assume that on the line AB shown in figure 12-18, you want to use a 100-ft tape to run a line from C perpendicular to AB. If a triangle with sides in the ratio of 3:4:5 is a right triangle, then one with sides in the ratio of 30:40:50 is also a right triangle. From C, measure off DC, 30 ft

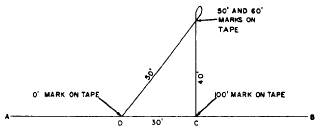
Figure 12-18.-Laying out a right angle using a 100-foot tape.

long. Set the zero-foot end of the tape on D and the 100-ft end on C. Have a person hold the 50-ft and 60-ft marks on the tape together and run out the bight. When the tape becomes taut, the 40-ft length from C will be perpendicular to AB.

MEASURING AN ANGLE BY TAPE. There are two methods commonly used to determine the size of an angle by tape: the CHORD method and the TANGENT method. The chord method can be applied, using the example shown in figure 12-19. Suppose you want to determine the size of angle A. Measure off equal distances from A (80.0 ft), and establish points B and C. Measure BC; assume that it measures 39.5 ft, as shown. You can now determine the size of angle A by applying the following equation:

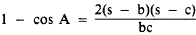
in which

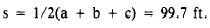
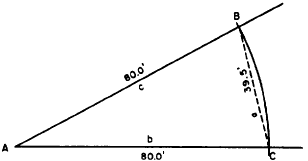
Figure 12-19.-Determining the size of an angle by the chord method.

First, solving for

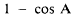
we have

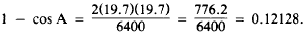
Since

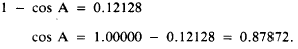
Reference to a table of natural functions shows that the angle with cos equal to 0.87872 measures, to the nearest 1 min., 2829.

The intervals measured off from A were made equal for mere convenience. The solution will work just as well for unequal intervals. In determining the size of an angle by the tangent method, you simply lay off a right triangle and solve for angle A by the common tangent solution.

Suppose that in figure 12-20, you want to determine the size of angle A. Measure off AC a convenient length (say, 80.0 ft). Lay off CB perpendicular to AC and measure it; say it measures 54.5 ft, as shown. The angle is computed by using the following formula:

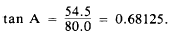
The angle with tangent 0.68125 measures 3418.