PRACTICE PROBLEMS:

Evaluate the following integrals:

ANSWERS:

METHOD 3

In the third method for solving integrals of quotients, we find that to integrate an algebraic function with a numerator that is not of lower degree than its denominator, we proceed as follows: Change the integrand into a polynomial plus a fraction by dividing the denominator into the numerator. After this is accomplished, apply the rules available.

EXAMPLE: Evaluate

SOLUTION: Divide the denominator into the numerator so that

Integrating each term separately, we have

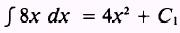
and

and

Then, by substitution, we find that

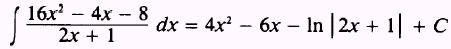
where

EXAMPLE: Evaluate

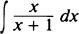
SOLUTION: The numerator in not of lower degree than the denominator; therefore, we divide and find that

Integrating each term separately, we find that

and

Therefore,

where

PRACTICE PROBLEMS:

Evaluate the following integrals:

ANSWERS: